How to Make a 5 Circuit Octagonal Labyrinth

May 3, 2022

Sometimes you need to talk about failure. Today I will do just that. I have done almost a dozen different step by step instructions on How to Make a Labyrinth. And I set out to add some additional content by adding an octagonal version to add to the classical, square and circular versions.

My first step was making the labyrinth myself. I sat down and did it….slowly and with many starts and stops. Do you know how to draw an octagon with equal sides ? I looked into it. Once I drew the first labyrinth I knew the second would be easier, and it was…a little bit. Then I drew another, and another. And finally I figured out how to easily give you step by step instructions on how to make the octagonal labyrinth ! Except for me it has still not become easy. Maybe a few more designs and I’ll figure it out completely.

So why are we here ? Well I’m going to show you what I learned to help you make your own. First we start with how to make an octagon !

How to Draw an Octagon - Method 1

If you want to draw an octagon with equal lengths here is the method I would use.

Step 1 Draw a straight line

Step 2 Rotate the line 45 degrees

Obviously this is a digital method where you can copy and paste then rotate, but it also works if you are hand drawing. Use a pencil and protractor/ruler and ensure you use the same length line.

Step 3/4 Rotate the line twice more at 45 degrees

This will create what looks like an asterisk. Or a cut pizza that has no crust (maybe I’m hungry?)

4 lines forming an asterix — Step 3 & 4 Rotate 45 degrees again

Step 5 Connect the ends of the lines

This will create outer walls of the octagon that are the same length.

how to make an octagon using lines — Connect the ends of the lines

Step 6 Delete the original lines & (optional) rotate

After deleting the guidelines, rotate the octagon 22.5 degrees so you have the bottom side flat on the page. I will mention that if you are drawing an octagonal labyrinth you may want to keep the lines to help guide you drawing that (explanation later in the post).

How to Draw an Octagon - Method 2

Alternatively you can also use a grid to draw an octagon that is “eyeballed” and has sides that are not quite equal. I will help you some with the math of that and show you how to get your sides very close. We start with the Isosceles Right Angle Triangle. On the left we have the formulas to calculate the length of the long side of the triangle, or if you remember from your school days the hypotenuse (the square root of 2 multiplied times the length). On the example on the right if the length of the sides are 8 this means the hypotenuse of the triangle is 11.3 units in length.

Wait, why are we talking about this ? Because if you use a grid to make an octagon the distance between the diagonals should not be equal to the length of the sides.

Check out this overlay of our blue original equal side octagon with the red drawn using a grid that uses 4 grid blocks to draw the sides. Each diagonal is too long. The length of 4 grids used is 4 DIAGONAL grids which are different than 4 vertical / horizontal grid lengths (because of the math). So you need to calculate the estimated length of the diagonal.

I did the math for you to calculate what grid lengths work best. The chart is on the left. With walls of 5 unit length, the Isosceles Right Angle Triangle hypotenuse length is calculated as 7.07 units (close enough that I would use this to draw an octagon). I highlighted the 4 that would work best in yellow.

On the right I show how these calculations translate into drawing on a grid. You reverse the lengths from the chart to draw your sides. So a 7 unit grid length is paired with a 5 grid diagonal (which is actually a length of 7.07). Similarly a 10 unit length paired with a 7 grid diagonal (actually length 9.9) also works. So using this math you can draw your own octagonal using grids and 45 degree angles.

I had planned on showing a second example comparing an octagon draw using the first method with one drawn using the second and the 5/7 grid calculation. When I aligned them they were virtually exact overlaps. So it works. Now we have our basic shape.

So everything is smooth sailing from here then ? Right ? No. Aligning the internal pathways correctly can best be described as trial and error. Why ? Because once you have your octagon drawn the internal walls might not line up the way you want with the grid you are working on.

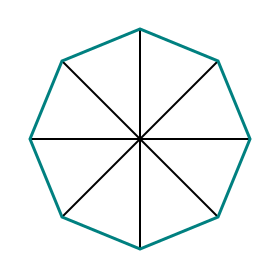
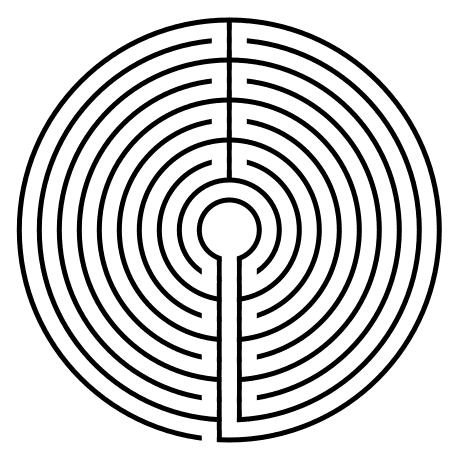
I wanted to draw an octagonal labyrinth with the walls and pathways of equal width at an equal width apart (similar to a standard equal maze if you are familiar with that construction). I think it looks best visually. If you are not concerned with that aesthetic you should be able to eyeball your labyrinth together much easier. Here is a standard equal labyrinth that I made that has 4 sections (4 internal turn-backs).

If you are eyeballing the drawing of this type of labyrinth, I suggest you start with the center goal portion and work your way out in layers. Could you design in the opposite direction, from the outside in ? Of course you can ! BUT, you may run out of room for your center goal ! If you go inside out, you only run out of room if you are using a piece of paper that is too small (and digitally you would never run out of room) !!

Also I want you to notice where the walls of the labyrinth make their turns. I have highlighted them below in red - If you made your Octagon using Method 1 above you will notice that the walls turn at the exact point it touches the line used to draw the octagon !! Keep this in mind as you draw your labyrinth ! You may also want to keep the original guides with this in mind.

5 circuit octagonal labyrinth showing how it is constructed

Normally, when I give step by step instructions I give specific advice and directions that work to draw an object (relatively) easily. Today, I give you instructions that will work, but will take some trial and error on your part !

How to Draw an Octagon Labyrinth - Method 1

This method can de done digitally or with a writing utensil. If you are drawing digitally and can “erase” I suggest you start with method 2.

Step 1 Draw a Center Octagon (The Goal)

Now that you know how to draw a good octagon let’s get started drawing our labyrinth with one that will create our goal. There is only 1 alteration that you need to make. In the bottom side of the labyrinth, leave a gap in the center for the final pathway. In my example that gap is equal to the width of the line I used to make my octagon.

IMPORTANT: From now on the examples will be formed in the following way: Black - previously drawn sections. Blue - current section you are creating. Red - future sections.

Step 2 Draw the first layer from the goal

This layer, shown in blue below consists of what look like brackets on each side of the center. Mine are an equal distance from the initial octagon. However you’ll notice that there are gaps at the top and bottom of the brackets. How do you know where these should start and stop ? For the top of the octagon, draw a centered line equal to 3X width of your walls. The brackets will end 1X width length away from that line (this creates your turnaround point). For the bottom section draw 2 lines down from the entrance to the goal also 3X width of the walls. Again your brackets will end 1X width away from them.

Step 3 Draw the second layer from the goal

This set of brackets is on a north south orientation. To determine where these end, draw a line centered on the right/left side of the previous bracket that measures 2X width. End brackets 1X width away from the these lines. Also, because of the pathway to the goal you will also need to leave a gap in the center of the bottom bracket !

Step 4 Draw the next layer

We return to the side brackets. We extend our north and south lines by 2X width. We extend and connect our left and right centered lines.

Step 5 Draw the next layer

North and south brackets. Extend the left and right centered lines.

Step 6 Draw the final outer edge

This final step has some changes to note. In the bottom section of the labyrinth, the the final pathway leading to the goal finally turns into the labyrinth. The only gap is the entrance, directly to the right of the final pathway goal wall. Also note that there is no extension of the wall at due north. This completes the drawing of the octagonal labyrinth.

Here is the final version with all lines in black.

A note about size. You can add additional steps if you would like, just continually adding internal sections until you are ready to finish the labyrinth by adding a final edge with an entrance.

A note about turn-backs. My example above has 4 sections. But you can also draw the same labyrinth with more or less turn-backs (sections). Below I have a 1 section, 2 section, 4 section (the one we just made) and 8 section version. If you imagine walking these, the more sections, or turn-backs, the more back and forth walking you will do.

5 circuit octagonal labyrinths with 4 different sections

How to Draw an Octagon Labyrinth - Method 2

The following method works for digital constructions. It uses a draw and cut construction. It is entirely written for brevity.

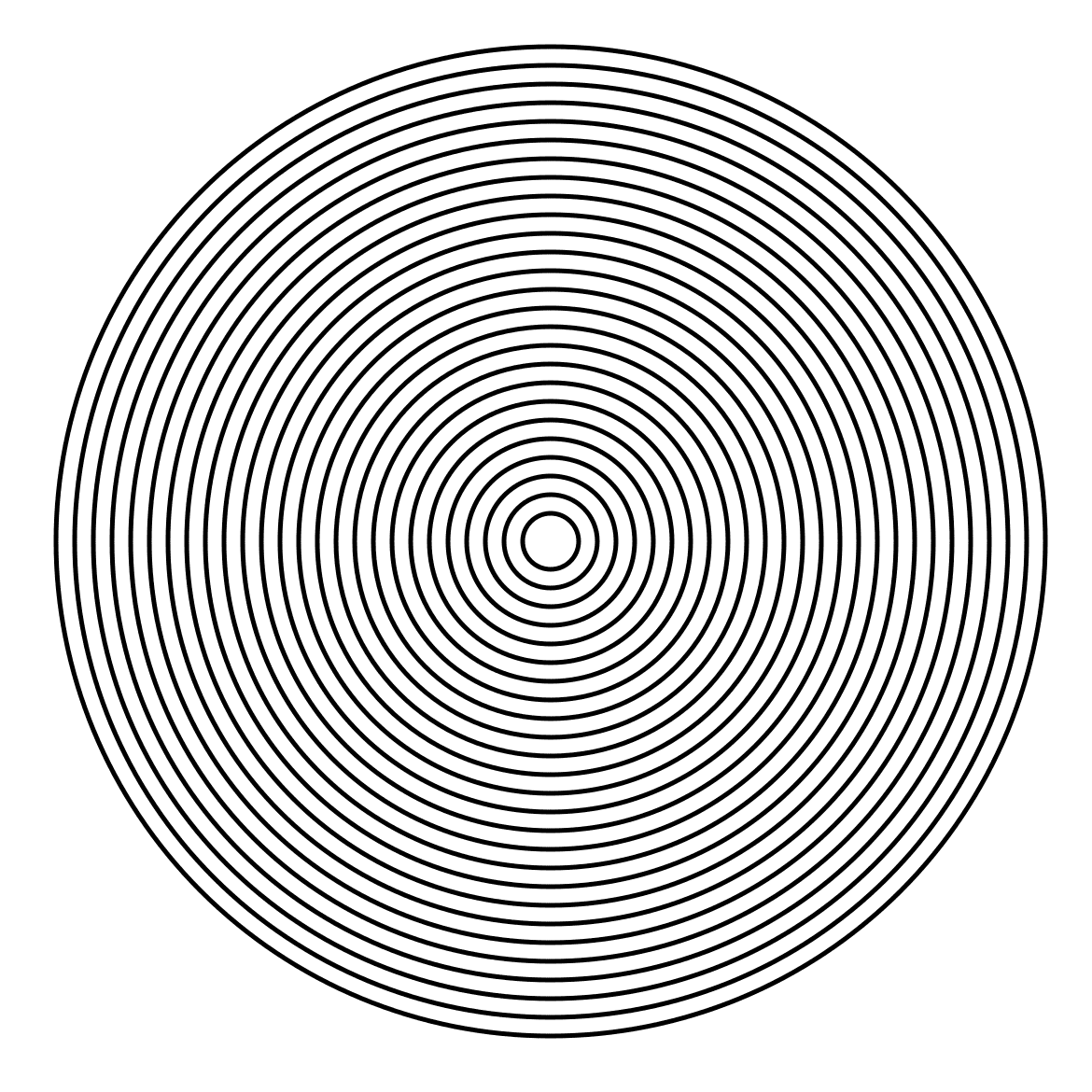
Step 1 - Draw 6 concentric octagons

Step 2 - Add turnback sections

Once you select how many turn-backs you will have ( my above example has 4 ), draw them, which will typically need one additional line than the # you choose to account for the centered bottom pathway that leads to the goal. The exception is a 1 section labyrinth which needs only 1 turnback.

For a 4 section these will be drawn at 3, 6 , 9 and 12 o’clock. With the extra line occurring at 6 where you create a centered final pathway to the goal. Ensure the lines at 3 and 9 have the innermost pathway open, while 12 has the top pathway open.

Step 3 - Open pathways

At the location of the turn-backs you will need to create the appropriate pathways, along with the entrance and goal pathways. This will create the final labyrinth.

Additional posts you might like:

The 12 best maze/labyrinth movies of all time

The Louvre Maze

Blue Mustang Maze

A Comparison of Possible 9 Circuit Circular Labyrinth Constructions

December 3, 2020

Previously we looked at how to digitally make a 9 Circuit Circular Labyrinth. Then I compared the 11 circuit labyrinths in circular, classic and square constructions. All of those findings also relate to 9 circuits. Today I will look at a few possible constructions of a 9 circuit circular labyrinth.

Let’s start with speaking about a classical labyrinth, which has a standard construction that is used. Learning how to draw that construction is standard across any source you might find. But, for a circular labyrinth there is no standard construction. If it is circular and the path moves from the outer edge to an internal goal in one continuous path, you have made a labyrinth. So when drawing one, you can get creative !! Let’s look at what some variations might look like.

Single Turn 9 Circuit Circular Labyrinth

Let’s start by looking at what a single turnback 9 circuit labyrinth would look like. Walking this would consist of long pathways, each getting shorter on your way to the center. Visually, not much going on.

2 Section 9 Circuit Circular Labyrinth

And now a version with 2 turnback’s, essentially breaking the labyrinth into sides. Walking this is more interesting with long walks getting shorter until you switch sides, then getting longer with a final long walk to the center.

3 Section 9 Circuit Circular Labyrinth

A now a standard 9 circuit broken into 3 sections. This could also have the entrance and final stretch pathways centered at the goal. My example only shows the final stretch to the goal centered. A little bit of mathematics - this is the only version where the initial pathway enters all the way into the maze. This would be the case for any odd numbered section circular labyrinth (like a 5 or 7 section - neither example is shown - try it !)

4 Section 9 Circuit Circular Labyrinth

And now the labyrinth broken into quadrants. Notice how the crossover point between the sides of the labyrinth is on the outside (a variation to my how-to). This is the more standard construction. But that side switch can take place in multiple layers if you want. This is the cleanest look IMO.

8 Section 9 Circuit Circular Labyrinth

Here we have an 8 section labyrinth. Things are getting crowded but there is still nice symmetry. If you had to walk this I think you would find it difficult to be relaxed since you are making turns frequently.

12 Section 9 Circuit Circular Labyrinth

And finally the final labyrinth construction I made, a 12 sectioned version. This version would make me dizzy if I tried to walk it. Luckily I only drew it.

4 Section 9 Circuit Circular Labyrinth

Now let’s go back and look at the 4 quadrant variant I taught how to make previously. It is a variant because the outer paths turn only once while the inner paths turn 4 times. This variation or other layer switchbacks are possible on any circular labyrinth. Feel free to play around and make new designs as you create your own labyrinths.

Every single construction you saw today was a 9 circuit circular labyrinth. Here is a look at the 6 main versions together:

6 different 9 Circuit Circular Labyrinth Construction options

As you make your own circular labyrinth realize that you can make any of these or as I did, a mix a few of them together. Good luck and happy labyrinth making !!

If you prefer making a different labyrinth, I have a section of step by step labyrinth making instructions.

Interested in learning how to make or draw other types of digital mazes ? I have step by step instructions on how to make over 40 different maze types.

How to Draw a 25 Circuit Circular Labyrinth

November 23, 2020

Today we look at how to digitally make a 25 Circuit Circular Labyrinth. Spoiler alert - This is the same as a 17 circuit circular labyrinth just adding a few more rings. This method is a draw and cut method, meaning you will be making edits that remove lines you have previously drawn as you move thru the steps. When I started this project I wanted to see how large I could make a circular labyrinth. For me, at more than 25 my screen and guides were too difficult to use. That’s how we got here. Let’s get started.

Step 1: Draw 26 Concentric Circles

Draw 26 concentric circles each an equal distance between one another and centered around the middle circle. The middle circle will become the labyrinths goal. As you may note, whatever the number of circuits you want is add one and that is how many circles you need.

Step 2: Draw the 10 Inner Walls

I could break this into more steps but there are 10 straight lines that form inner walls and pathways. See the example. All occur at 3, 9, and 12 o clock or centered around 6 o’clock. Rather than write it out, follow the example. Imagine you are riding a subway system and mind the gaps.

Step 3: Add Pathways Where Needed

Refer to the picture and add pathways where needed, erasing portions of the circles. There are many, and depending on how you draw/erase them the number can change. They are all at 3, 6, 9 and 12 o’ clock, next to where you just drew inner walls.

That completes the drawing of a 25 Circuit Circular Labyrinth.

Interested in learning how to make or draw other types of digital mazes ? I have step by step instructions on how to make over 40 different maze types.

If you prefer making labyrinths, you can find step by step labyrinth making instructions.

How to Draw a 17 Circuit Circular Labyrinth

November 22, 2020

Today we look at how to digitally make a 17 Circuit Circular Labyrinth. This method is a draw and cut method, meaning you will be making edits that remove lines you have previously drawn as you move thru the steps. It is possible to use a drawing only method but I think it is much more complicated with many more steps. Let’s get started.

Step 1: Draw 18 Concentric Circles

Draw 18 concentric circles each an equal distance between one another and centered around the middle circle. The middle circle will become the labyrinths goal.

Step 2: Draw the 6 Inner Walls

I could break this into more steps but there are 6 straight lines that form inner walls and pathways. See the example. At 12 o’clock create 2 lines, blocking paths between all circles except 15-16. At 3 o’clock add a pathway wall to block circles between 2 and 16. Use 2 lines to draw a pathway from the center to the bottom edge (notice neither are at 6 o’clock). The right line will stop one circle short of the outer circle. Add a line at 9 o’clock blocking the pathways between circles 2 and 16.